Weiyang Lin
Design Optimization of Acoustic Metamaterials and Phononic Crystals With a Time Domain Method
A Dissertation Presented for the Doctor of Philosophy in Computational Engineering, The University of Tennessee at Chattanooga
Weiyang Lin, December 2016
Abstract:
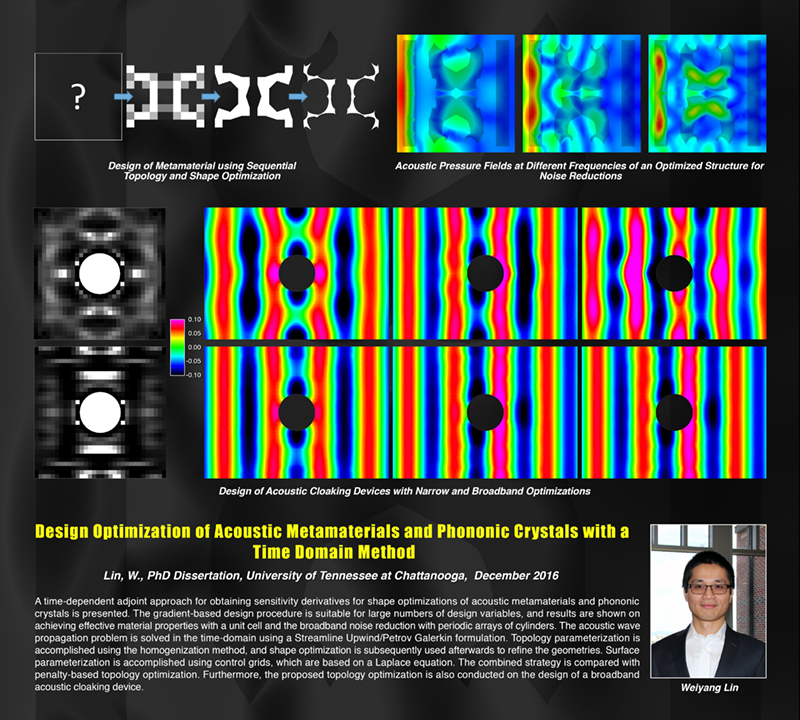
A time-dependent adjoint approach for obtaining sensitivity derivatives for shape optimizations of acoustic metamaterials and phononic crystals is presented. The gradient-based design procedure is suitable for large numbers of design variables, and results are shown on achieving effective material properties with a unit cell and the broadband noise reduction with periodic arrays of cylinders. The acoustic wave propagation problem is solved in the time-domain using a Streamline Upwind/Petrov Galerkin formulation. Topology parameterization is accomplished using the homogenization method, and shape optimization is subsequently used afterwards to refine the geometries. Surface parameterization is accomplished using control grids, which are based on a Laplace equation. The combined strategy is compared with penalty-based topology optimization. Furthermore, the proposed topology optimization is also conducted on the design of a broadband acoustic cloaking device.
Click here to access a copy of Weiyang's dissertation.