Lawton Shoemake
Extension of A High-Order Petrov-Galerkin Implementation Applied to Non-Radiating and Radar Cross Section Geometries
A Thesis Presented for the Master of Science in Computational Engineering Degree, The University of Tennessee at Chattanooga
W. Lawton Shoemake, December 2013
Abstract:
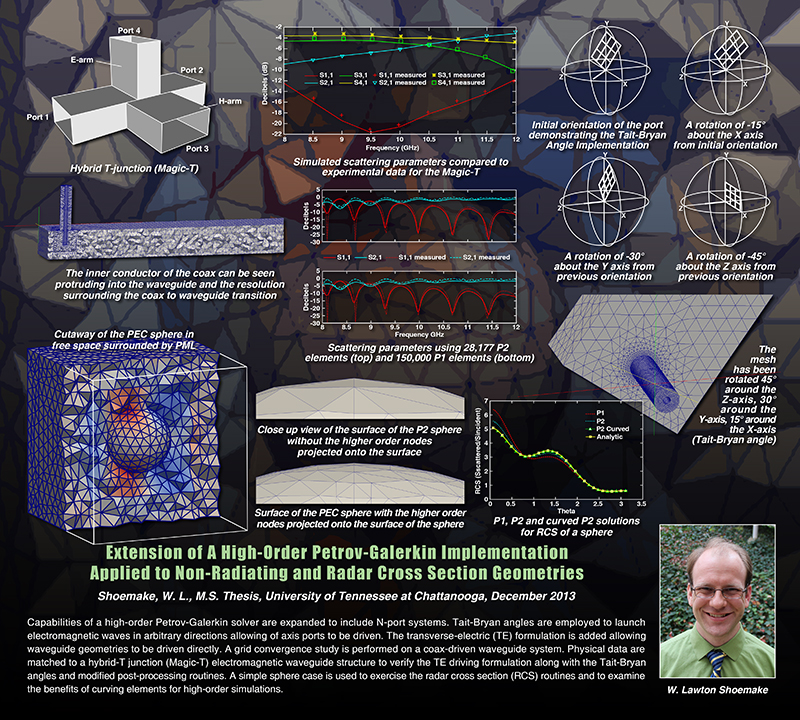
Capabilities of a high-order Petrov-Galerkin solver are expanded to include N-port systems. Tait-Bryan angles are employed to launch electromagnetic waves in arbitrary directions allowing of axis ports to be driven. The transverse-electric (TE) formulation is added allowing waveguide geometries to be driven directly. A grid convergence study is performed on a coax-driven waveguide system. Physical data are matched to a hybrid-T junction (Magic-T) electromagnetic waveguide structure to verify the TE driving formulation along with the Tait-Bryan angles and modified post-processing routines. A simple sphere case is used to exercise the radar cross section (RCS) routines and to examine the benefits of curving elements for high-order simulations.
Click here to access a full copy of Lawton's thesis.