Srijith Rajamohan
A Streamline Upwind/ Petrov-Galerkin FEM Based Time-Accurate Solution of 3D Time-Domain Maxwell's Equations for Dispersive Materials
A Dissertation Presented for the Doctor of Philosophy in Computational Engineering, The University of Tennessee at Chattanooga
Srijith Rajamohan, August 2014
Abstract:
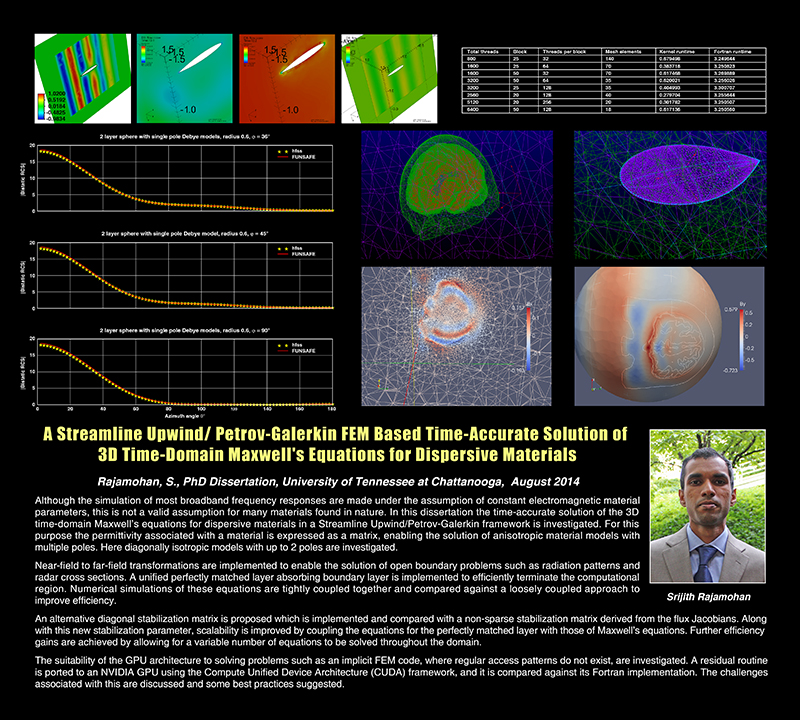
Although the simulation of most broadband frequency responses are made under the assumption of constant electromagnetic material parameters, this is not a valid assumption for many materials found in nature. In this dissertation the time-accurate solution of the 3D time-domain Maxwell’s equations for dispersive materials in a Streamline Upwind/Petrov-Galerkin framework is investigated. For this purpose the permittivity associated with a material is expressed as a matrix, enabling the solution of anisotropic material models with multiple poles. Here diagonally isotropic models with up to 2 poles are investigated.
Near-field to far-field transformations are implemented to enable the solution of open boundary problems such as radiation patterns and radar cross sections. A unified perfectly matched layer absorbing boundary layer is implemented to efficiently terminate the computational region. Numerical simulations of these equations are tightly coupled together and compared against a loosely coupled approach to improve efficiency.
An alternative diagonal stabilization matrix is proposed which is implemented and compared with a non-sparse stabilization matrix derived from the flux Jacobians. Along with this new stabilization parameter, scalability is improved by coupling the equations for the perfectly matched layer with those of Maxwell’s equations. Further efficiency gains are achieved by allowing for a variable number of equations to be solved throughout the domain.
The suitability of the GPU architecture to solving problems such as an implicit FEM code, where regular access patterns do not exist, are investigated. A residual routine is ported to an NVIDIA GPU using the Compute Unified Device Architecture (CUDA) framework, and it is compared against its Fortran implementation. The challenges associated with this are discussed and some best practices suggested.
Click here to access a copy of Srijith's dissertation.